Power
&
Effect Size
Overview
- Review & Elaboration of Hypothesis Testing
- Power
- Effect Size
Review & Elaboration of Hypothesis Testing
What Hypothesis Is Being Tested
- Research questions are often couched in terms of an “alternate”
hypothesis (HA) that there is some difference
- E.g., that a mean is different than some value (e.g., the mean is not zero)
- Or that there is a difference between two means
- (Ignore for now that other times we’re testing not a difference, but a relationship)
What Hypothesis Is Being Tested (cont.)
- But we actually test the viability of a “null” hypothesis
(H0)
- E.g., the probability of finding our sample mean if the population mean is actually zero
- Or the probability of finding our mean difference if there really is no true difference between the groups
- So, the p-value we report is the probability of finding our results if the null hypothesis is true
E.g., Testing Against H0 = 0
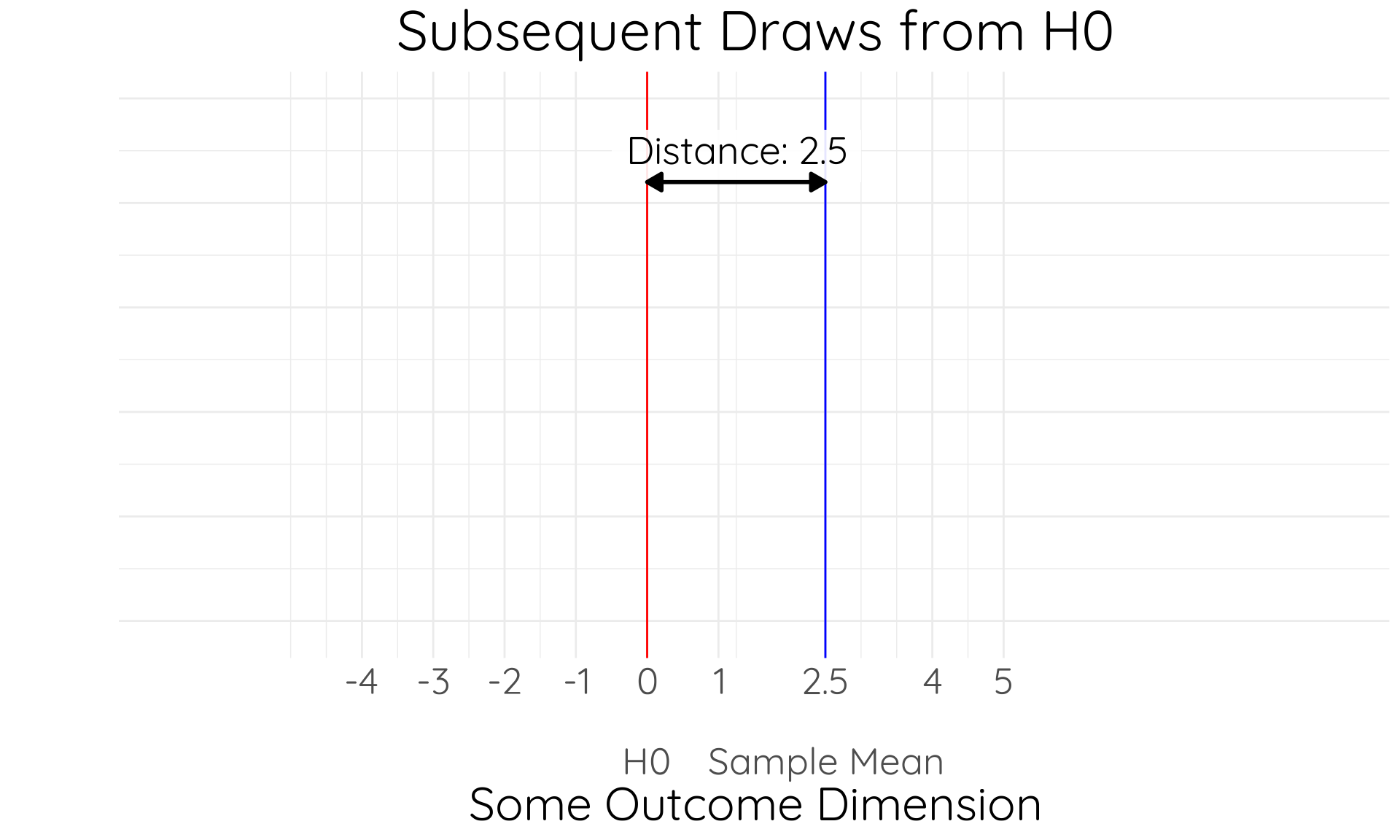


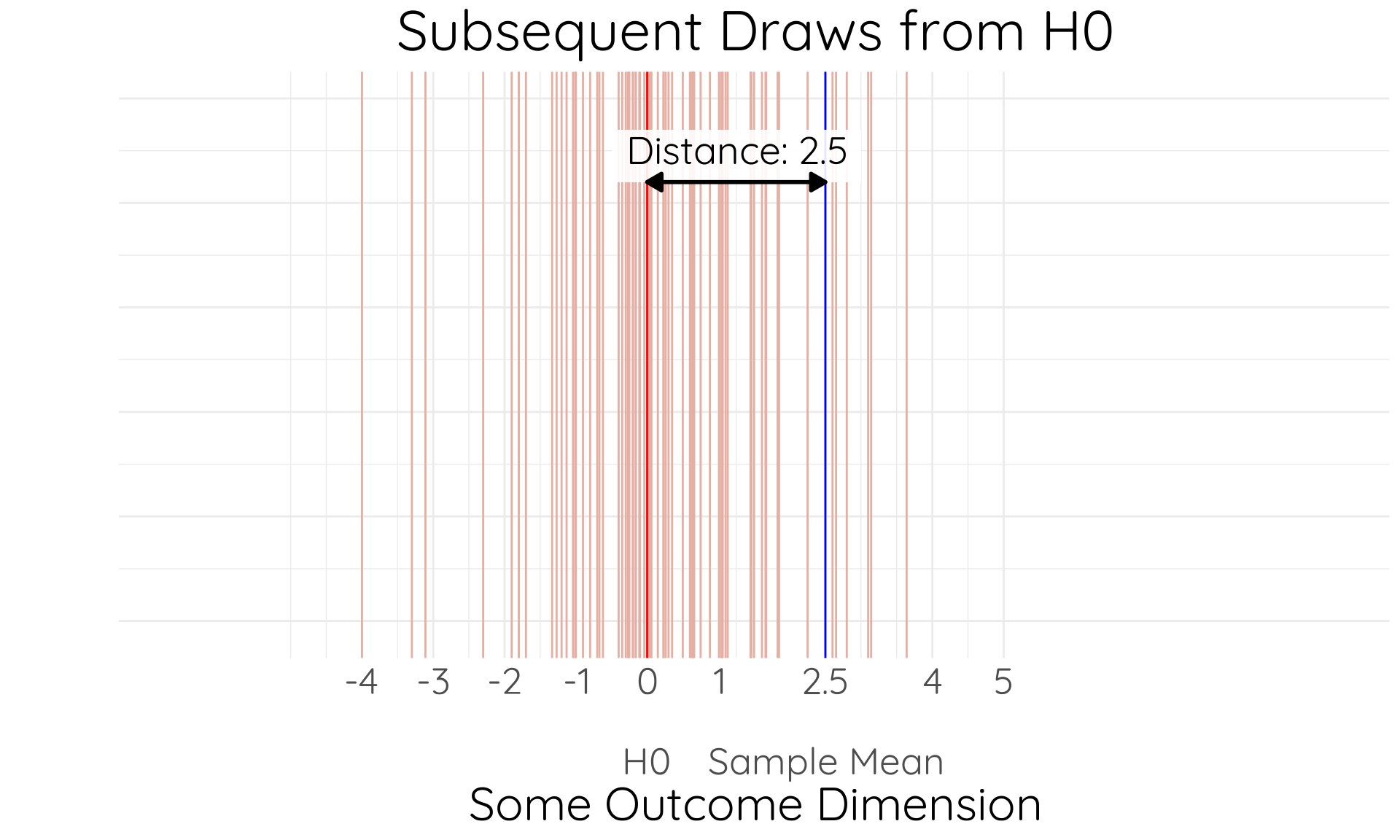
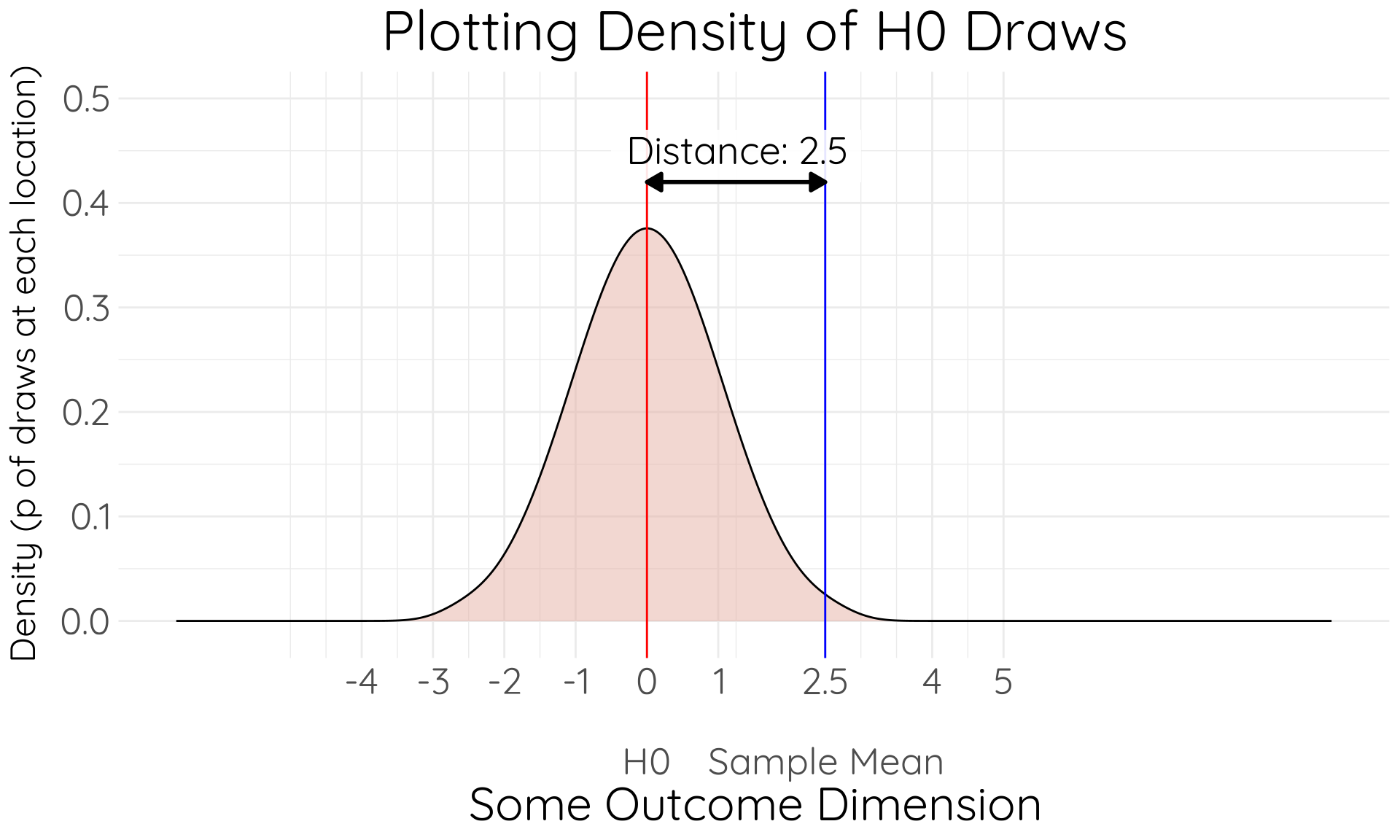

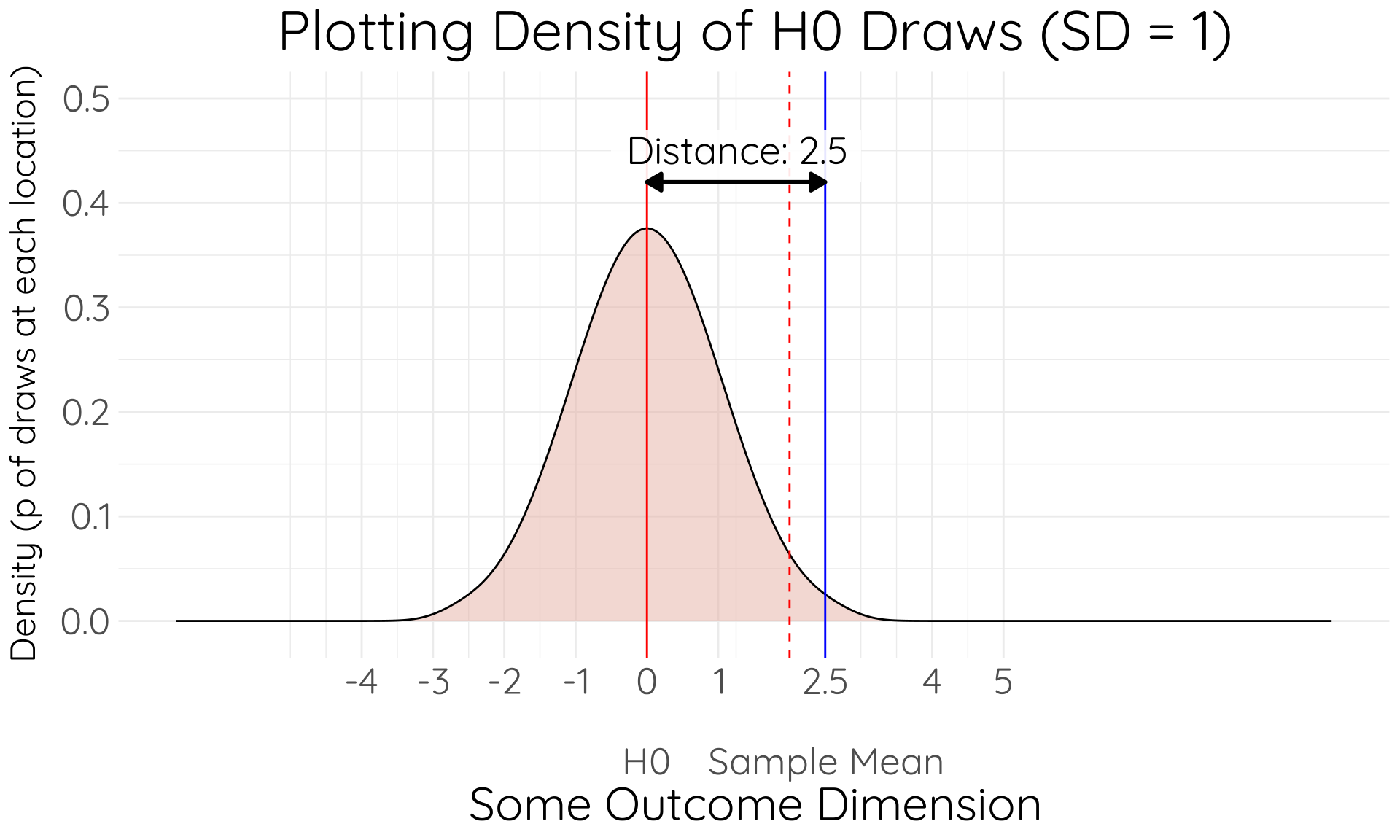
Testing Against an
Other H0
Distribution
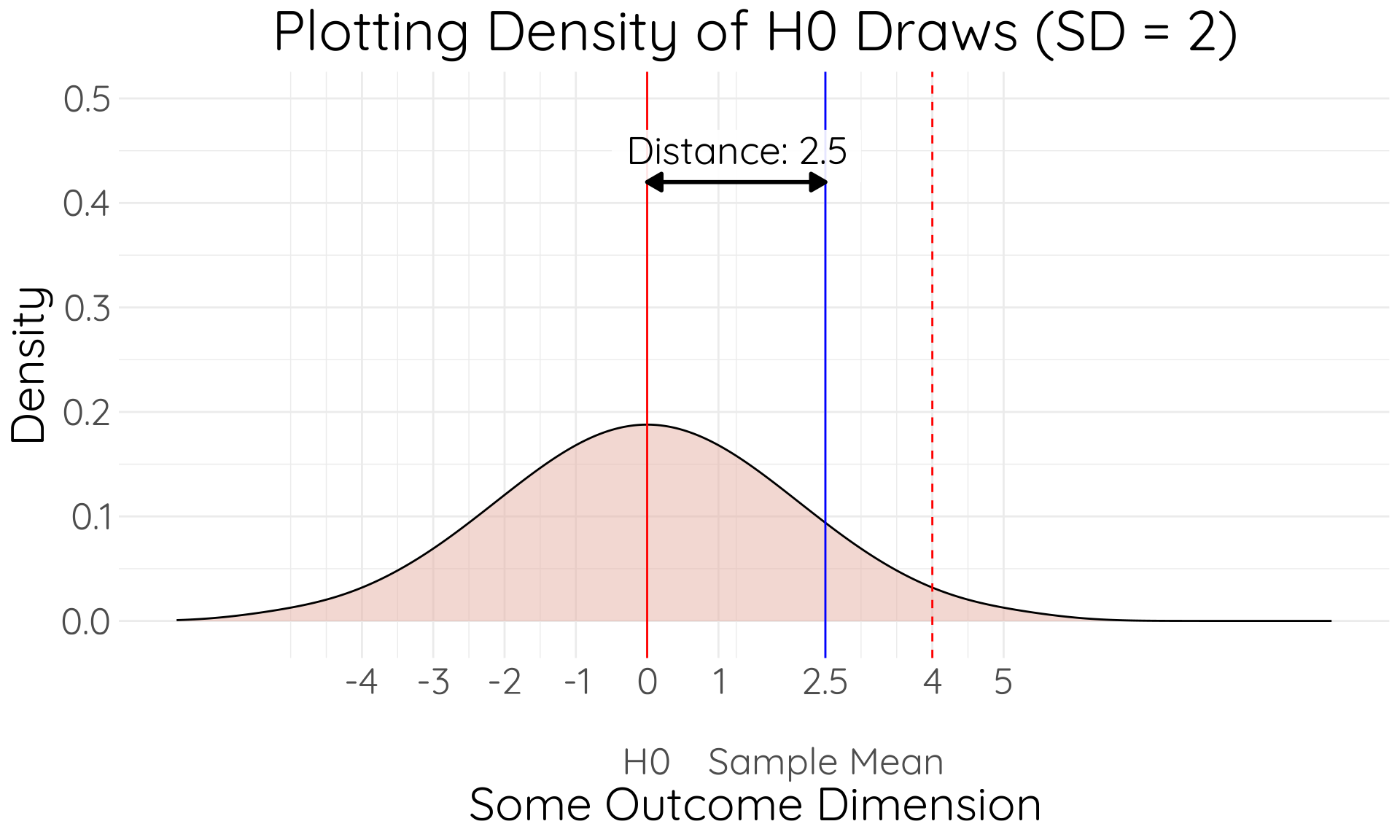
Testing Between Two Distributions
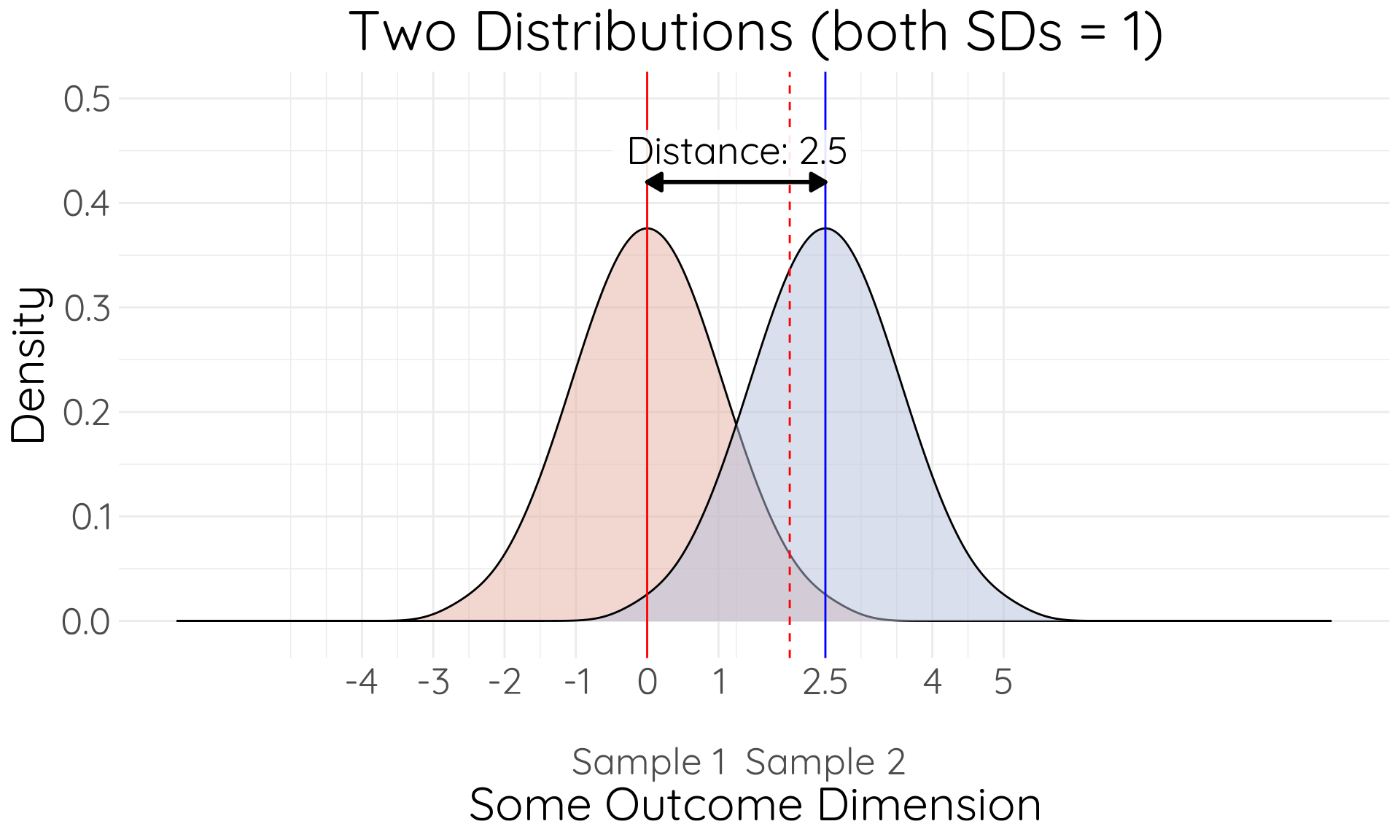
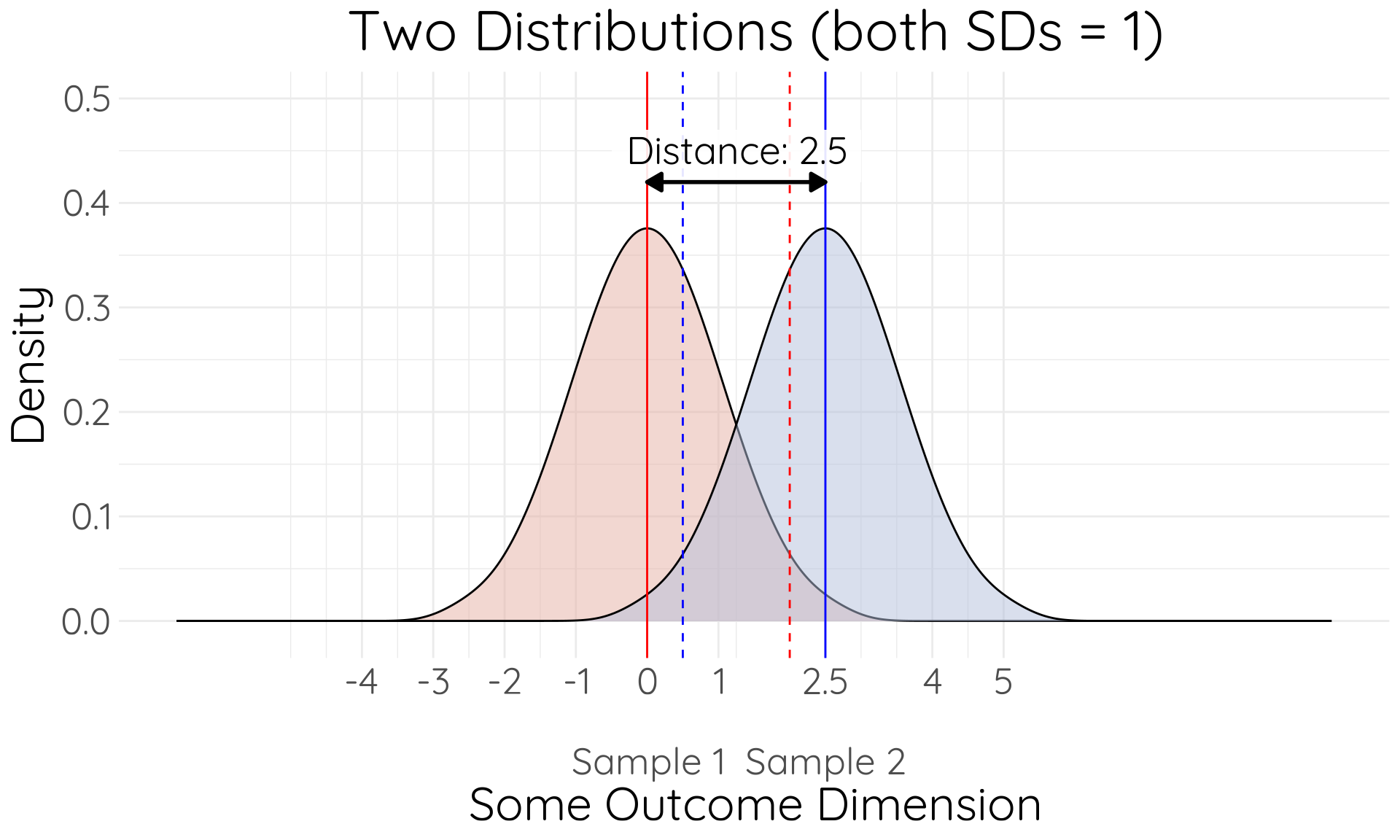
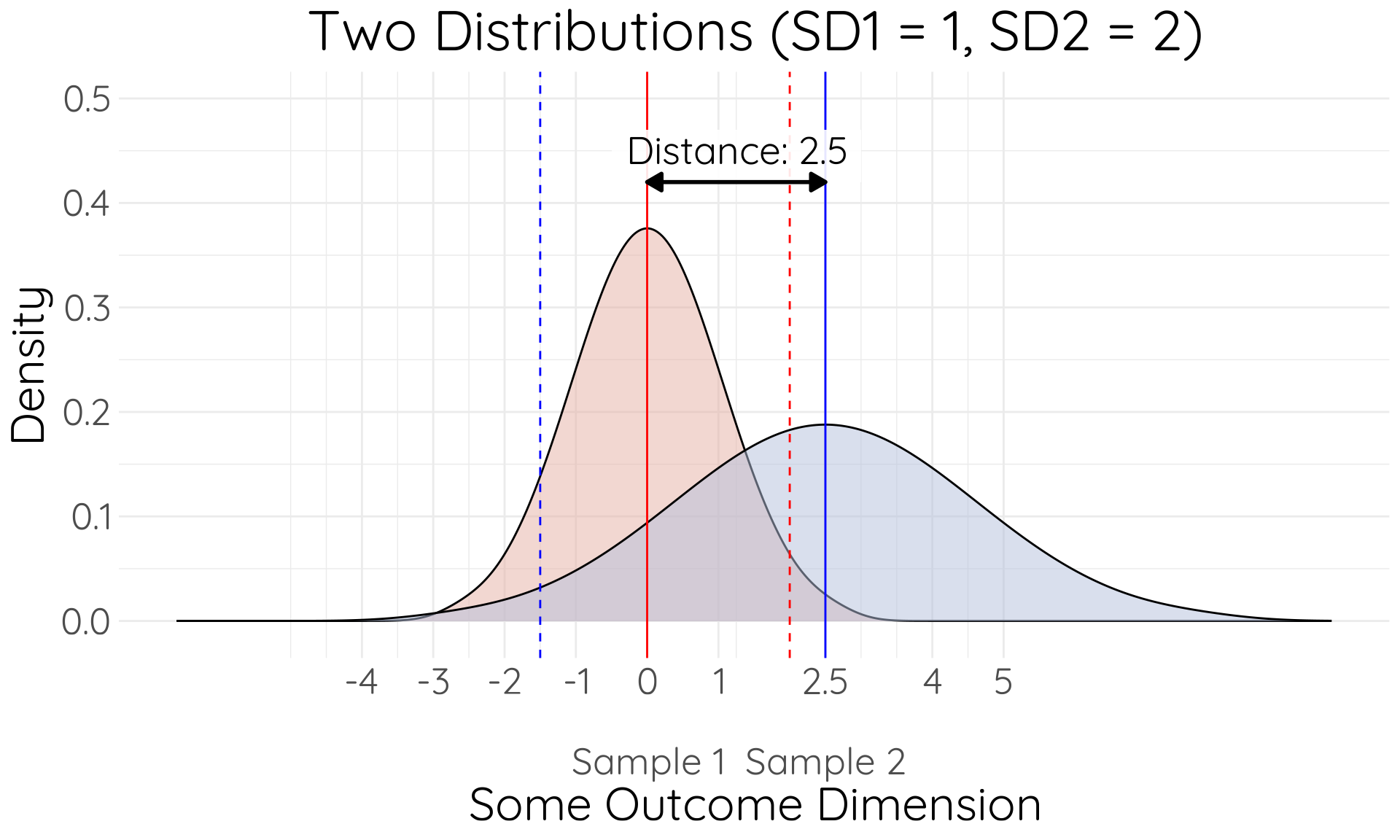
Couched as Signal & Noise
How can we improve our chances of correctly finding a real difference?
1. Increase the Signal
How do we increase the signal?
Increasing the Signal
- We can best increase the signal through good research design:
- Improve the effectiveness of our intervention
- Investigate impactful things
- Measuring—and thus being able to detect—a wide range of outcomes
- Including among a wide range of participants/situations
2. Reduce the Noise
How do we reduce the noise?
Reducing the Noise
- Improve measurement precision
- Reduce the noise from imprecise measurements
- More reliable instruments/measurements
- Make finer measurements—not just more accurate
- Reduce the noise from imprecise measurements
- Improve sampling techniques
- Controlled data collection situations
- Careful use of instructions, ordering of items/instruments
- Consideration of sources of bias
- And reasons for missing data / refusal to answer—or even participate
Reducing the Noise (cont.)
2. Improve sampling techniques (cont.)
- Ensure it is a well-delimited population
- Diverse in ways that improve “good” variance
- Unitary in respects to other sampling criteria
- (Essentially “measuring” the population well—clearly and across its full range)
3. Control for sources of noise
- Measuring & managing
- Accounting for similarities between participants, situations, etc.
Reducing the Noise (end)
4. Increase sample size
- Remember, these distributions are of estimates of the population
values
- Viz., the estimates of the population mean
- And the “noise” in these estimates is the standard error of the mean
- The formula for the SEM is:
- Viz., the estimates of the population mean
\[ SEM = \frac{SD}{\sqrt{N}} \]
- So, we can reduce “noise” by increasing the sample size
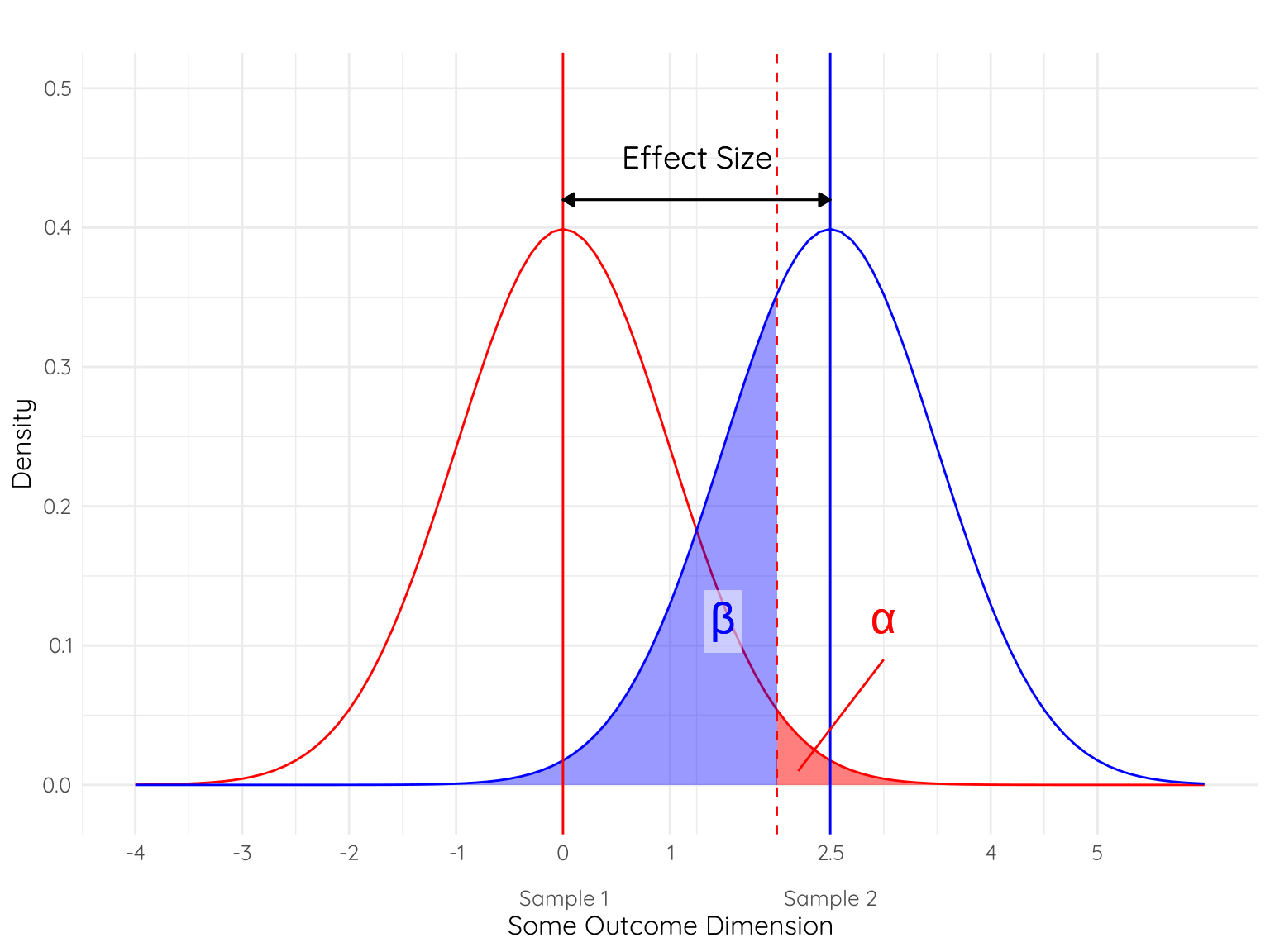
Power
Understanding Power
- Conceptually, power is our ability to see things clearly
- A lot like increasing the magnification of a microscope
Understanding Power (cont.)
“The power of a statistical test is the probability that it will yield statistically significant results.” (Cohen, 1988, p. 1)
“The power of a statistical test of a null hypothesis is the probability that it will lead to the rejection of the null hypothesis,
- i.e., the probability that it will result in the conclusion that the phenomenon exists.” (p. 4)
Understanding Power (cont.)
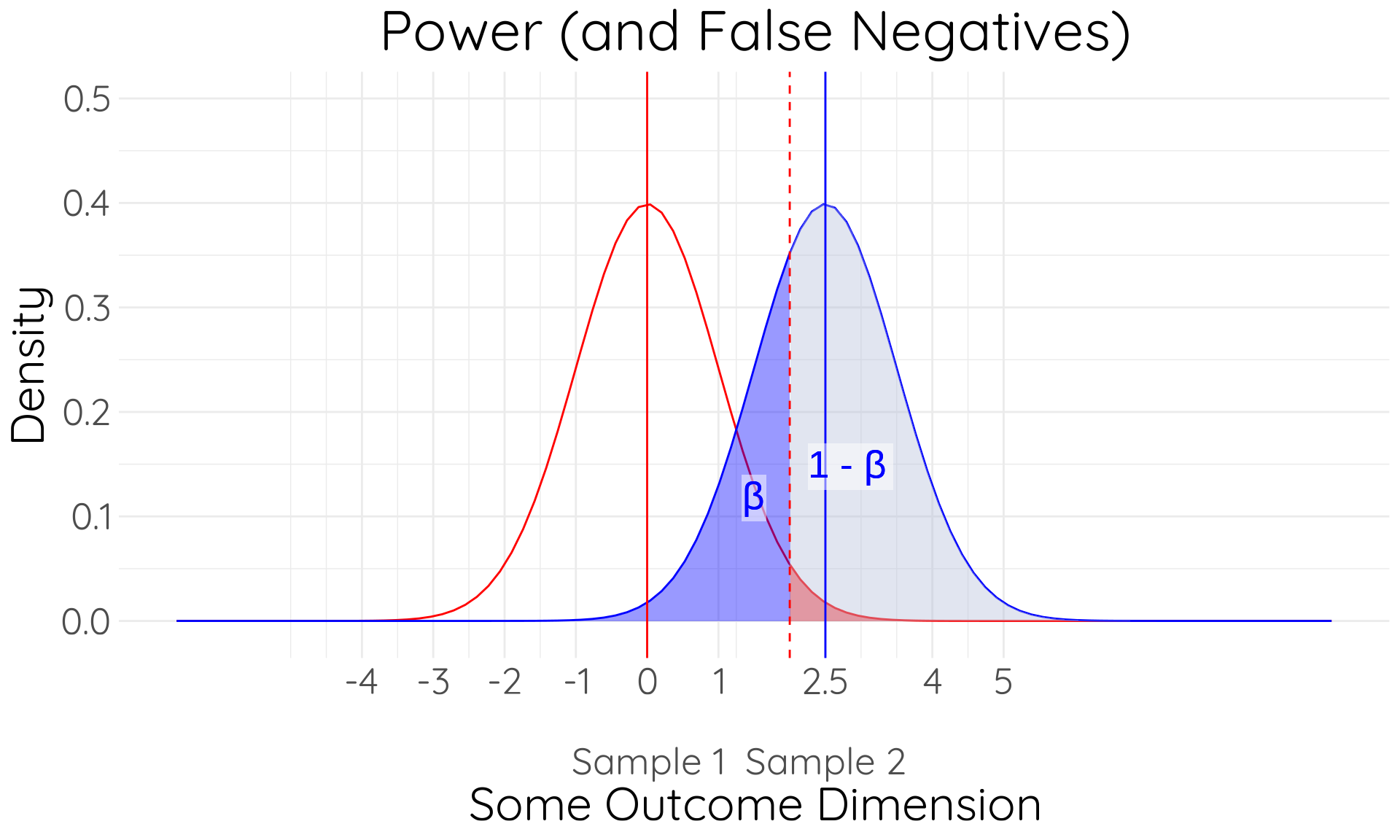
- In hypothesis testing, a lack of power is the
probability of getting a false negative
- I.e., the chance of missing an effect that actually is there
- High power means little chance of missing a real effect
- Low power means a real effect can easily be missed
- The probability of a false negative is written as β
- (To correspond with the chance of a false positive, which is written as α)
- So power—i.e., not missing a real
effect—is
1 – β
Understanding Power (cont.)
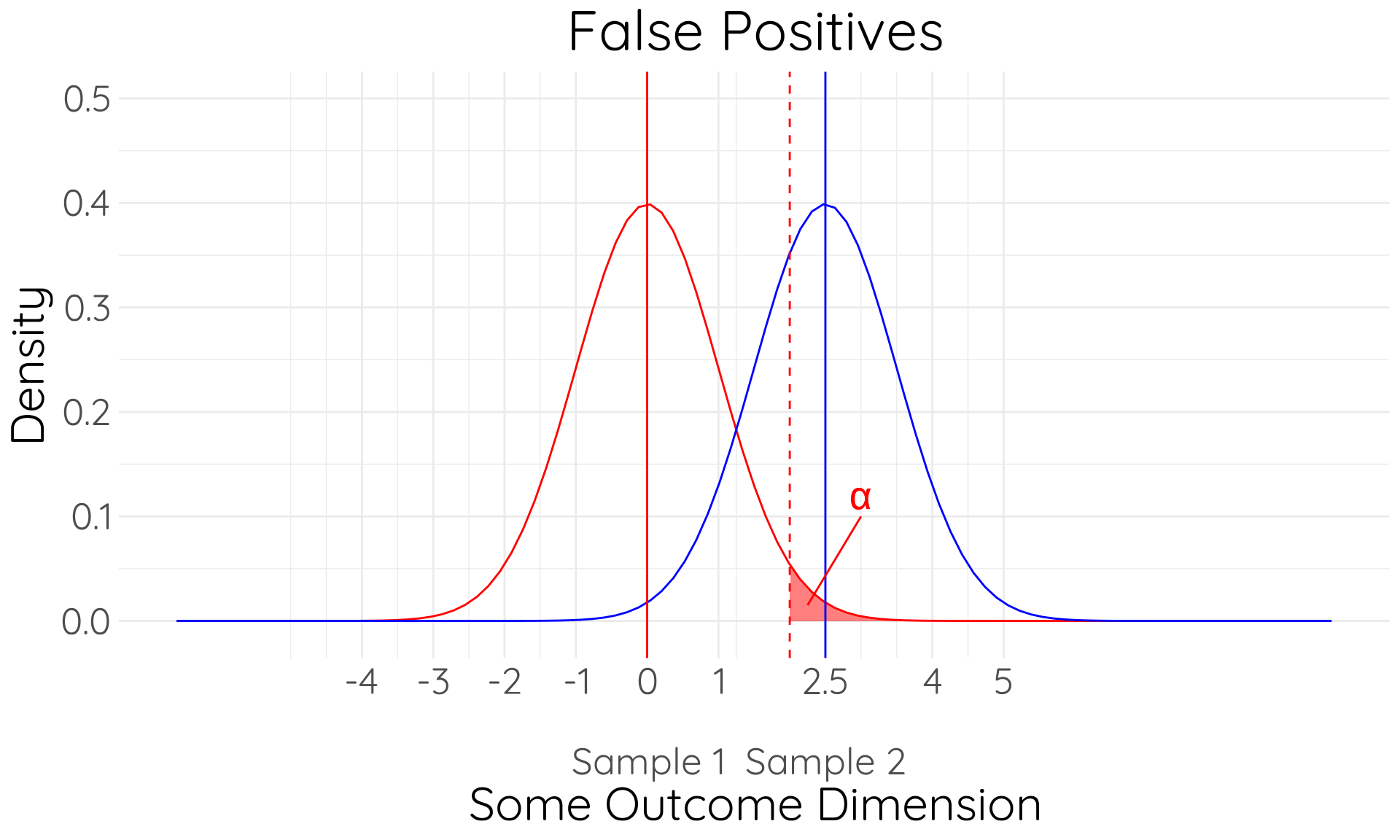

Understanding Power (end)

- Yes, a false positive (α) is also called a Type 1 error
- And a false
negative (β) is
also called a Type
2 error
- But everyone gets confused by that, so I just stick with “false positive” & “false negative”
Increasing Power
How can we increase power?
- Increase signal (also called “effect size”)
- Reduce noise
- Through good measurement, sampling & design, and accounting for unexplained variance
- By increasing sample size
- But also…
Changing Power by Changing α
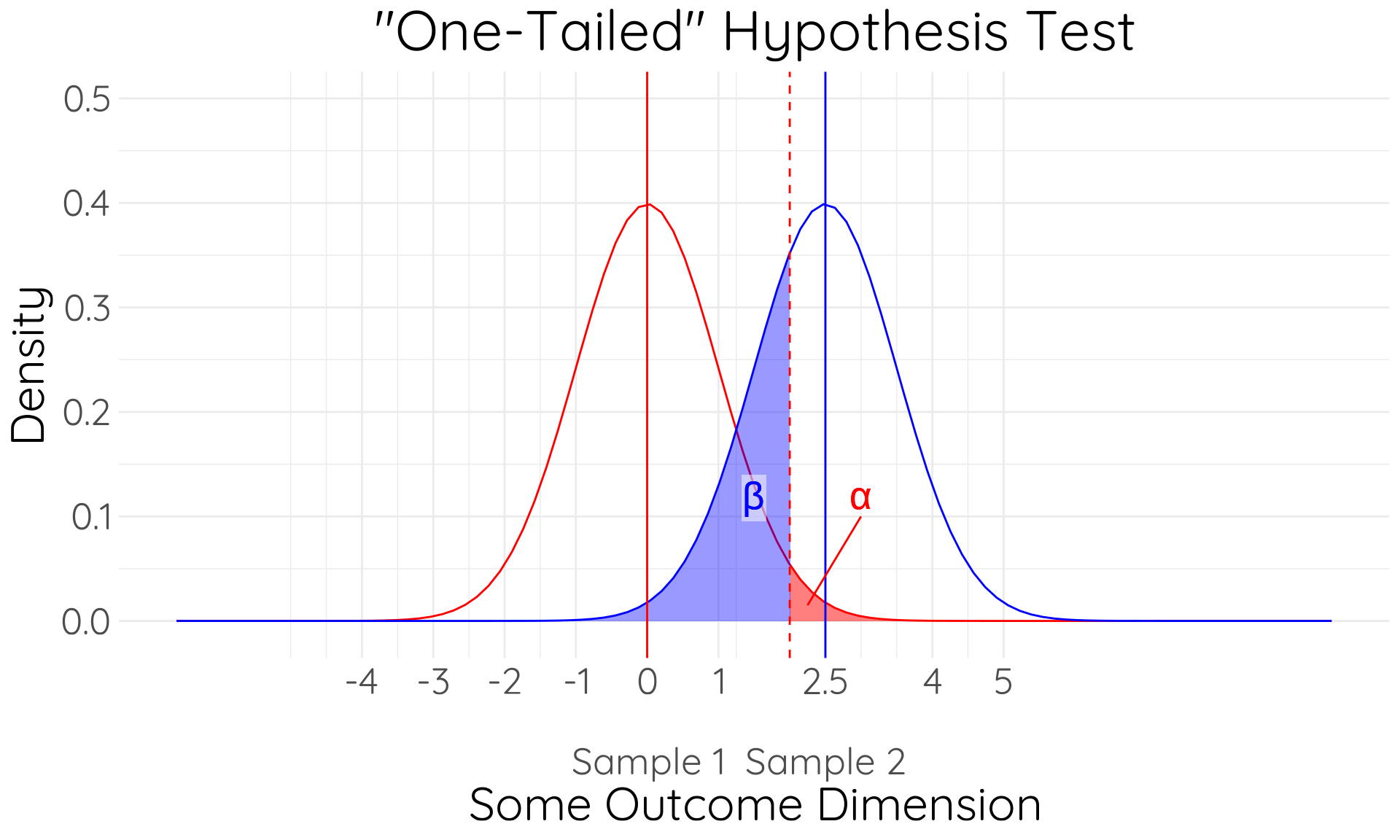
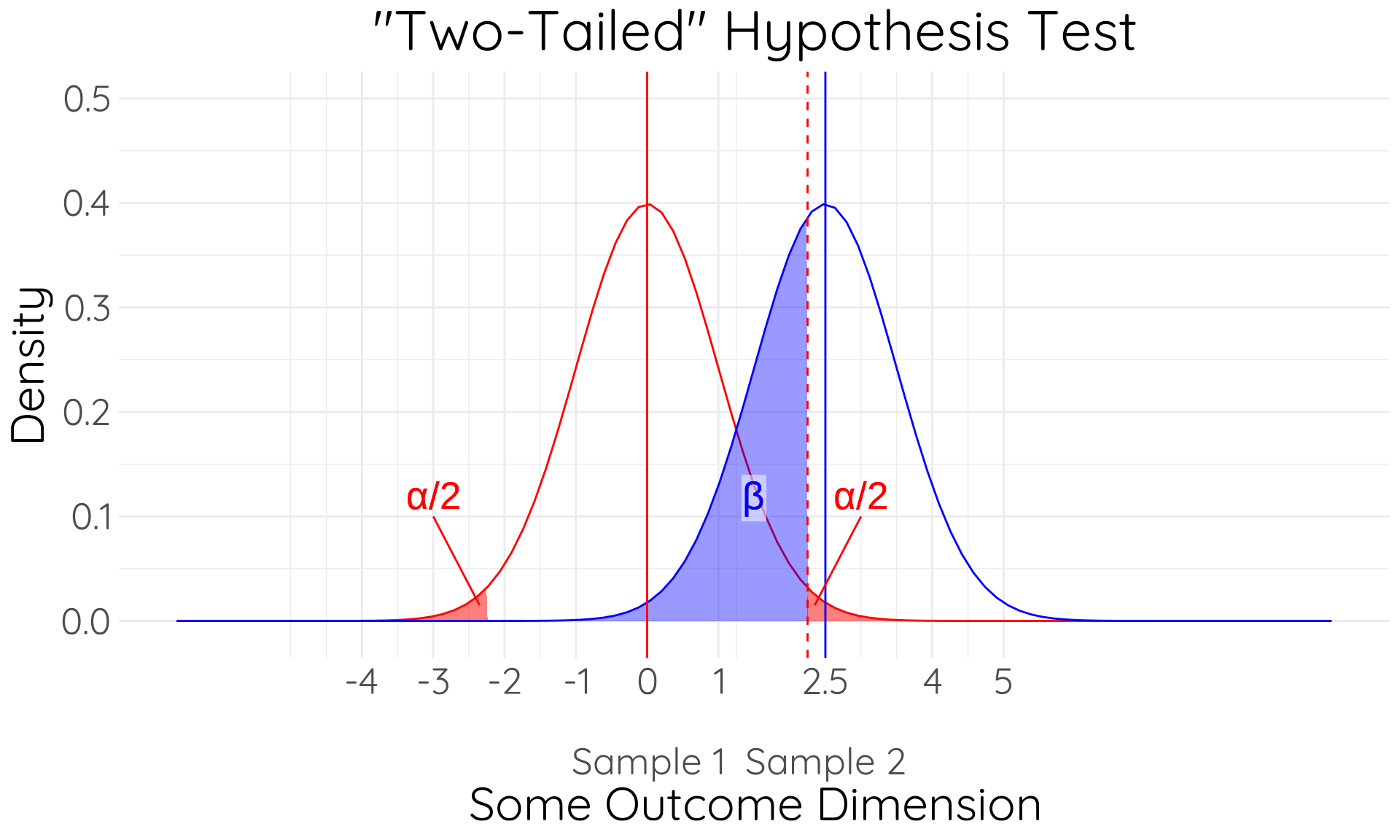
Changing Power by Changing α (cont.)
- One-tailed tests are more powerful than two-tailed tests
- But ask a more specific—restrictive—question:
- Two-tailed tests ask:
“Is there any difference between these groups?” - One-tailed tests ask:
“Is Sample 2 larger than Sample 1?”
- Two-tailed tests ask:
Changing Power by Changing α (end)
-
We generally use two-tailed tests
- They are more conservative
- Allow us to see even unexpected effects
- But we should use whichever is theoretically appropriate
- Remembering that with more power comes more responsibility
Post hoc Power Analysis
- Aka “observed” (or “retrospective”) power
- Remember:
- When conducting hypothesis tests, we are actually assuming the null
hypothesis (H0) is true
- E.g., α is the chance of a false positive—incorrectly assuming a sample value is not from H0
- While all the time still assuming that null hypothesis is true
- When conducting hypothesis tests, we are actually assuming the null
hypothesis (H0) is true
Post hoc Power Analysis (end)
- Power, then, is the chance of finding an effect
- Assuming the null hypothesis is true
- If we find significance, though, we know no longer assume the null
is true
- So conducting power analyses after hypothesis testing uses refuted assumptions
- Post hoc power is thus nearly always fallacious
- Post hoc power is only justified when the population values are know (e.g., O’Keefe, 2007)
- And thus can be computed based on that distribution(s) instead of the null
Effect Size
Definition
“‘[T]he degree to which the phenomenon is present in the population’ or ‘the degree to which the null hypothesis is false.’” (Cohen, 1988, pp. 9 – 10)
- The size of a difference
- Between groups, a different times, etc.
- Between the odds/risks of various groups, etc.
- E.g., higher odds ratio
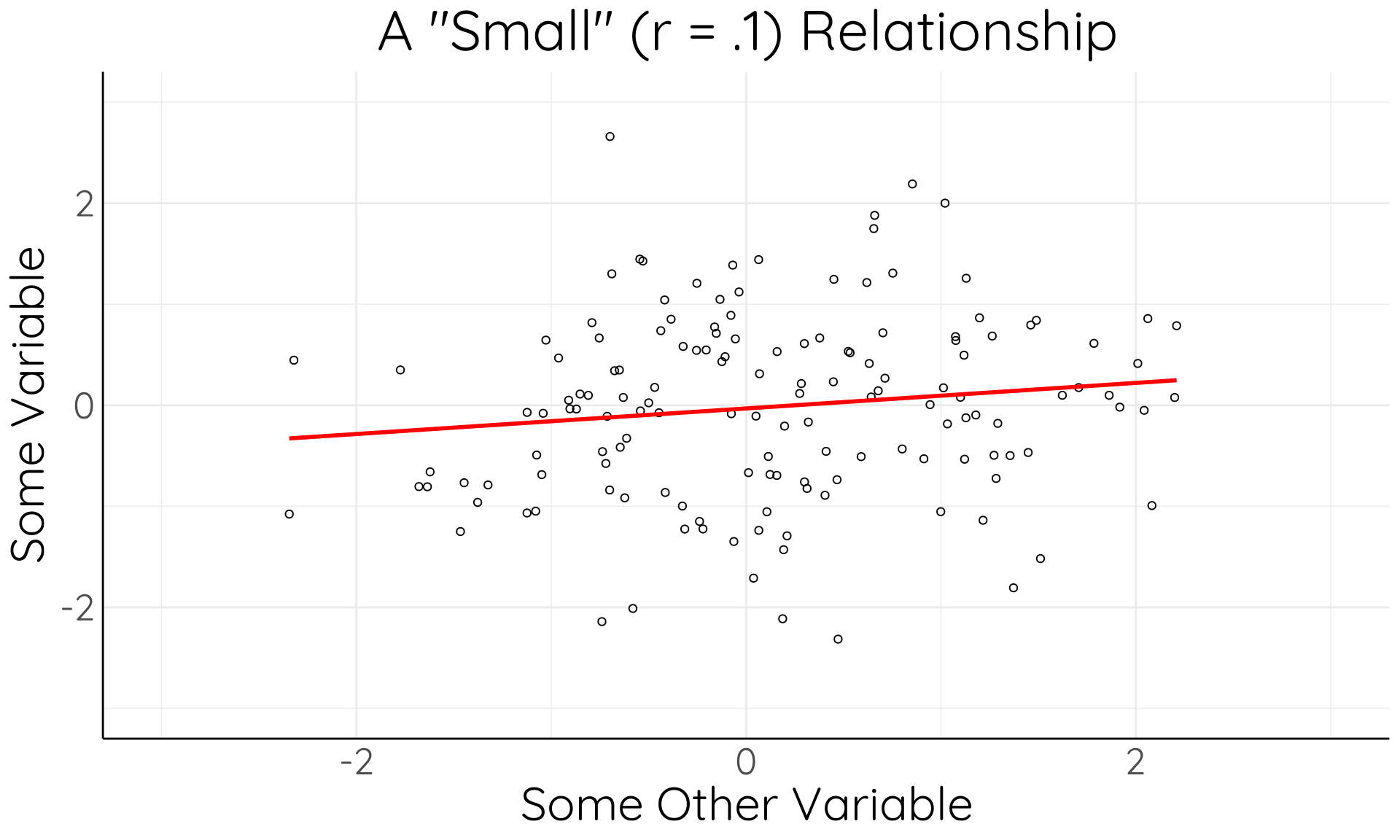
- The strength of relationships between variables
- Higher correlations
- The tendency for things to co-occur
- E.g., higher odds
Effect Size is the “Signal”
Effect Size Statistics
- Effect size statistics are standardized
- z scores
- I.e., SDs
- Proportions
- Correlations (proportions of variance accounted for)
- Odds ratios, risk/hazard ratios
- z scores
- We can thus compare a given effect size statistic across similar
studies
- (This is what is done in meta-analyses)
- However, we cannot directly compare two different effect size
statistics
- But we can convert them mathematically
Effect Size Statistics (cont.)
- We can also present effect sizes with, e.g., 95% confidence
intervals
- For differences and relationships,
- The effect is significant if the 95% CI doesn’t overlap zero
- For odds, risks, hazards (& their ratios)
- It’s significant if the 95% CI doesn’t overlap 1
- For differences and relationships,
- This can complement formal significance tests
- And also shows not only the size, but our, well, confidence in any conclusions made about it
Common Effect Size Statistics
| Type of Effect | Statistic |
|---|---|
| Difference between two means | Cohen’s d |
| Association between two variables | Correlation (r, rpb, φ, etc.) |
| Partial association between two variables | Cohen’s f & f\(^2\), η\(^2\) |
| Likelihood of co-occurrence | Odds, risks/hazards |
| Relative likelihood of co-occurrence | Odds ratios, risk/hazard ratios |
- More info, including a few less common effect size statistics, is in the Companion
Those Deuced Sizes
- I.e., whether an effect size statistic is “small,” “medium,” or “large”
- Cohen proposed values for each measure he reivewed/created
- Cohen never meant his criteria to be canonical
- But to serve “as a convention” while pointing out that “all
conventions are arbitrary” (p. 13)
- “The terms ‘small,’ ‘medium,’ and ‘large’ are relative, not only to each other, but to the area of behavioral science or even more particularly to the specific content and research method being employed in any given investigation” (p. 25)
- But to serve “as a convention” while pointing out that “all
conventions are arbitrary” (p. 13)
Those Deuced Sizes (cont.)
- “A reader who finds that what is here defined as ‘large’ is too small (or too large) to meet what [their] area of behavioral science would consider appropriate standards is urged to make more suitable operational definitions. (p. 79)
- “Thus, what a sociologist may consider a small effect size may well be appraised as medium by a clinical psychologist.” (p. 285)
- “Again, the reader is urged to avoid the use of these conventions, if [they] can, in favor of exact values provided by theory.” (p. 113)
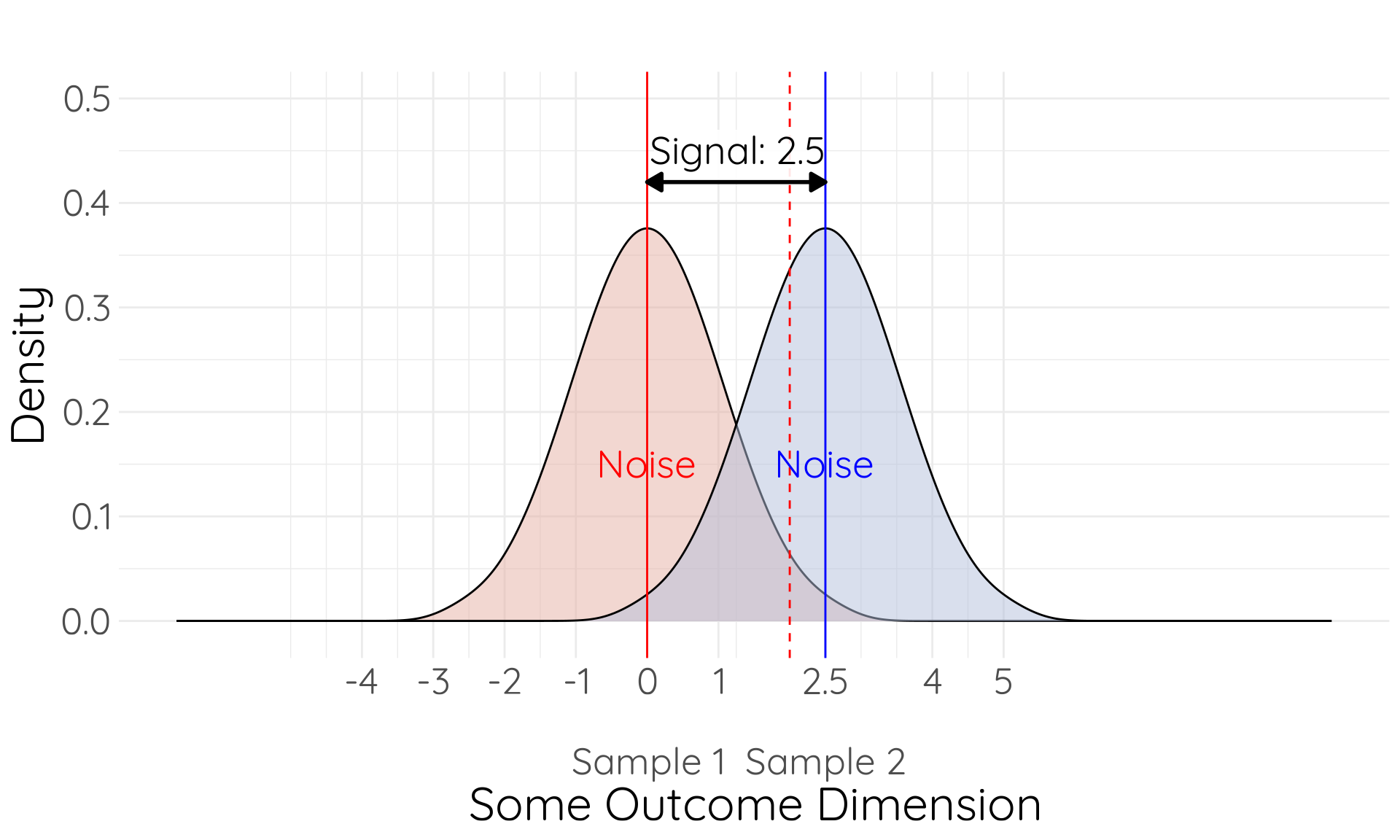
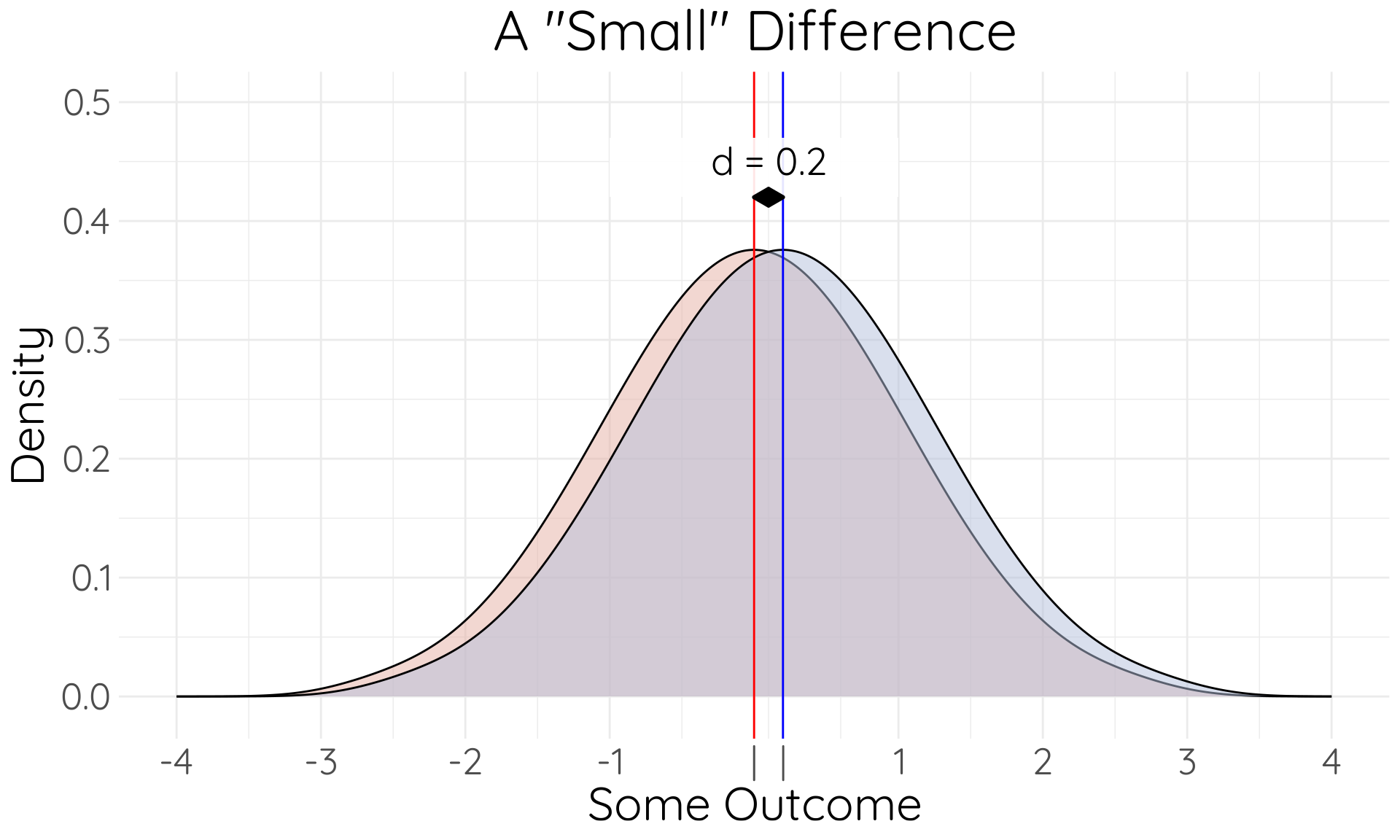
A “Small” Effect
- Small: “must not be so small that seeking them
amidst the inevitable operation of measurement and experimental bias and
lack of fidelity is a bootless task”
- The value was chosen in part because the “many relationships pursued in ‘soft’ behavioral science are of this order of magnitude” (p. 79)
A “Small” Effect (cont.)
A “Small” Effect (cont.)
A “Small” Effect (end)
- About 85% overlap between two distributions
- 1% of total variance accounted for (2% for R2)
- Detecting prevalences of 55:45 versus 50:50
- E.g.:
- Mean difference in IQ between twins and non-twins
- Approximately the size of the difference in mean height between 15- and 16-year-old girls (i.e., 0.5 in. where the σ \(\approx\) 2.1, p. 26)
- For significance, would expect to need¹ Ns of:
- t test: 394
- r: 782
¹ Assuming power (1 – β) = .8, α = .05, and normally-distributed samples.
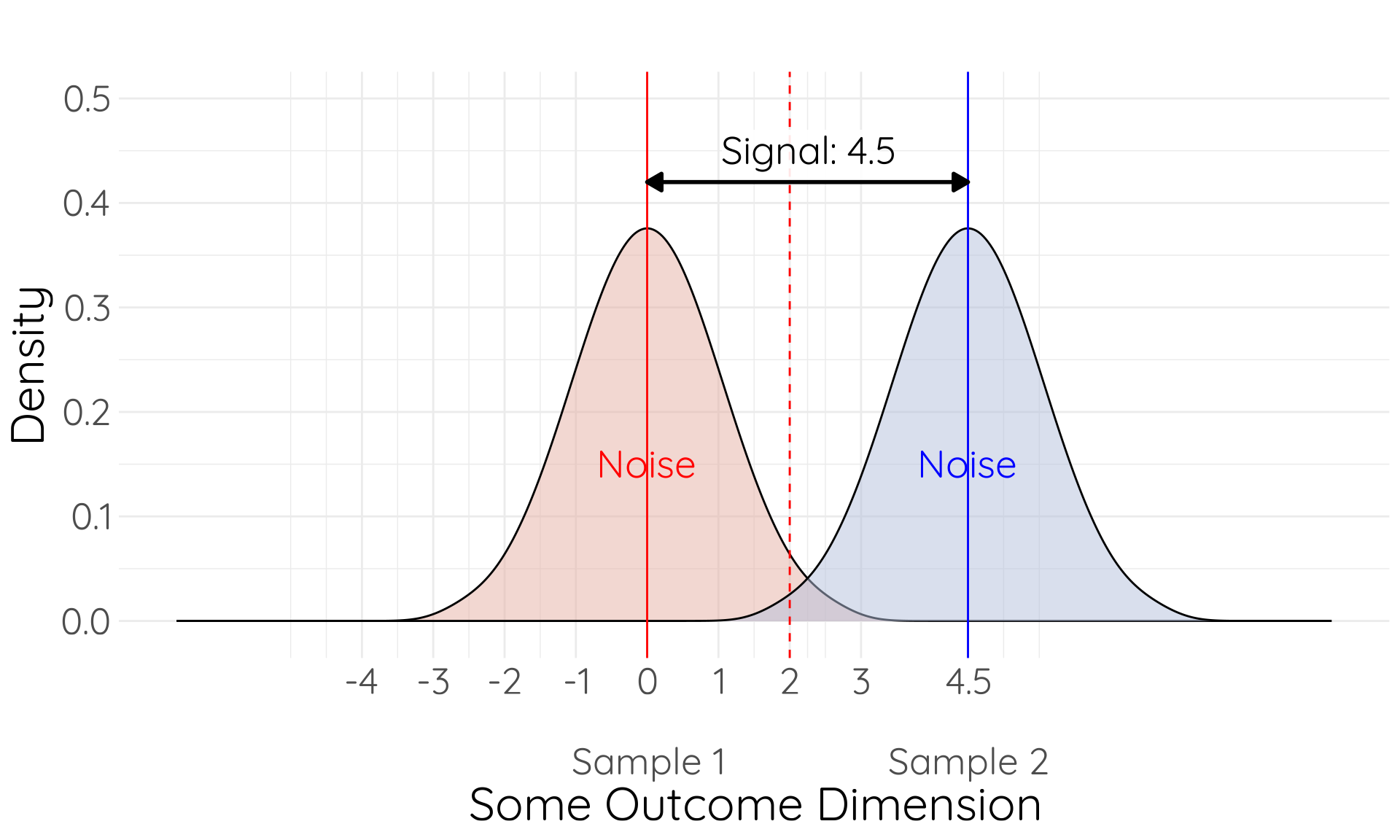
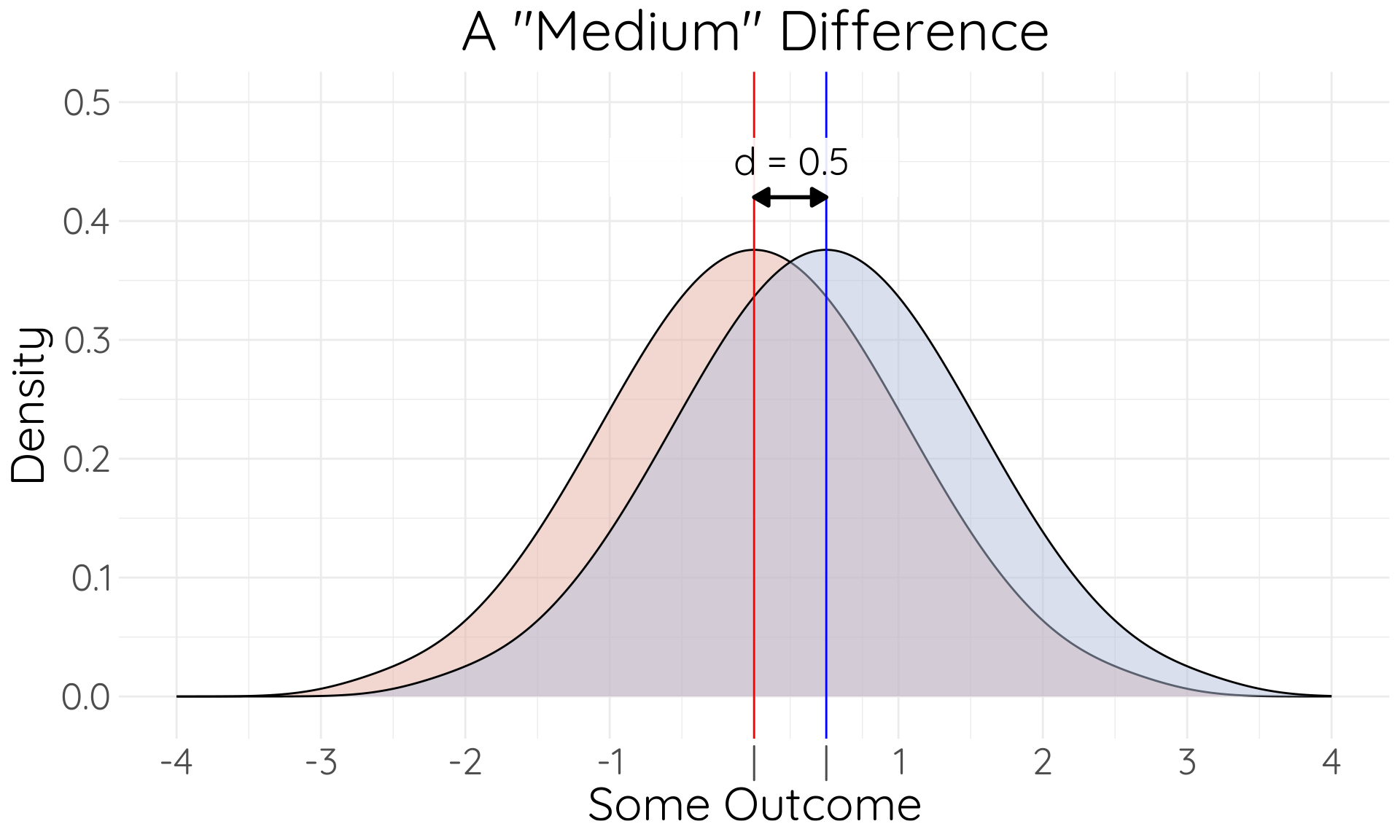
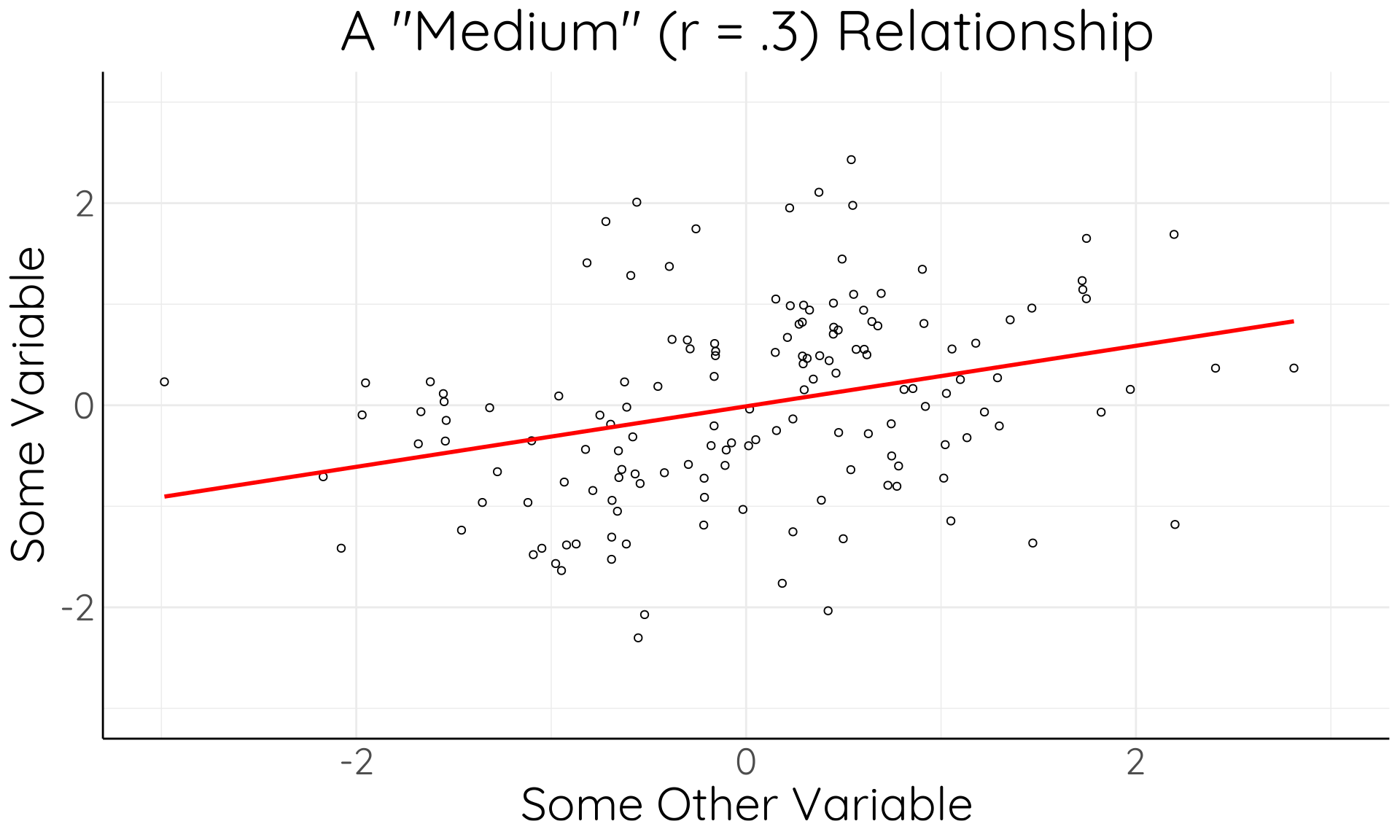
A “Medium” Effect
A “Medium” Effect (cont.)
A “Medium” Effect (cont.)
A “Medium” Effect (end)
- ~67% overlap between two distributions
- ~10% of total variance accounted for (13% for R2)
- Detecting prevalences of 65:35 versus 50:50
- E.g., a mean difference in IQ between members of clerical and
semi-skilled occupations
- Correlation between adolescents’ creativity & IQ
- Approximately the size of the difference in mean height between 14- and 18-year-old girls (i.e., 1 in. where the σ = 2)
- For significance, would expect to need Ns of:
- t test: 64
- r: 84
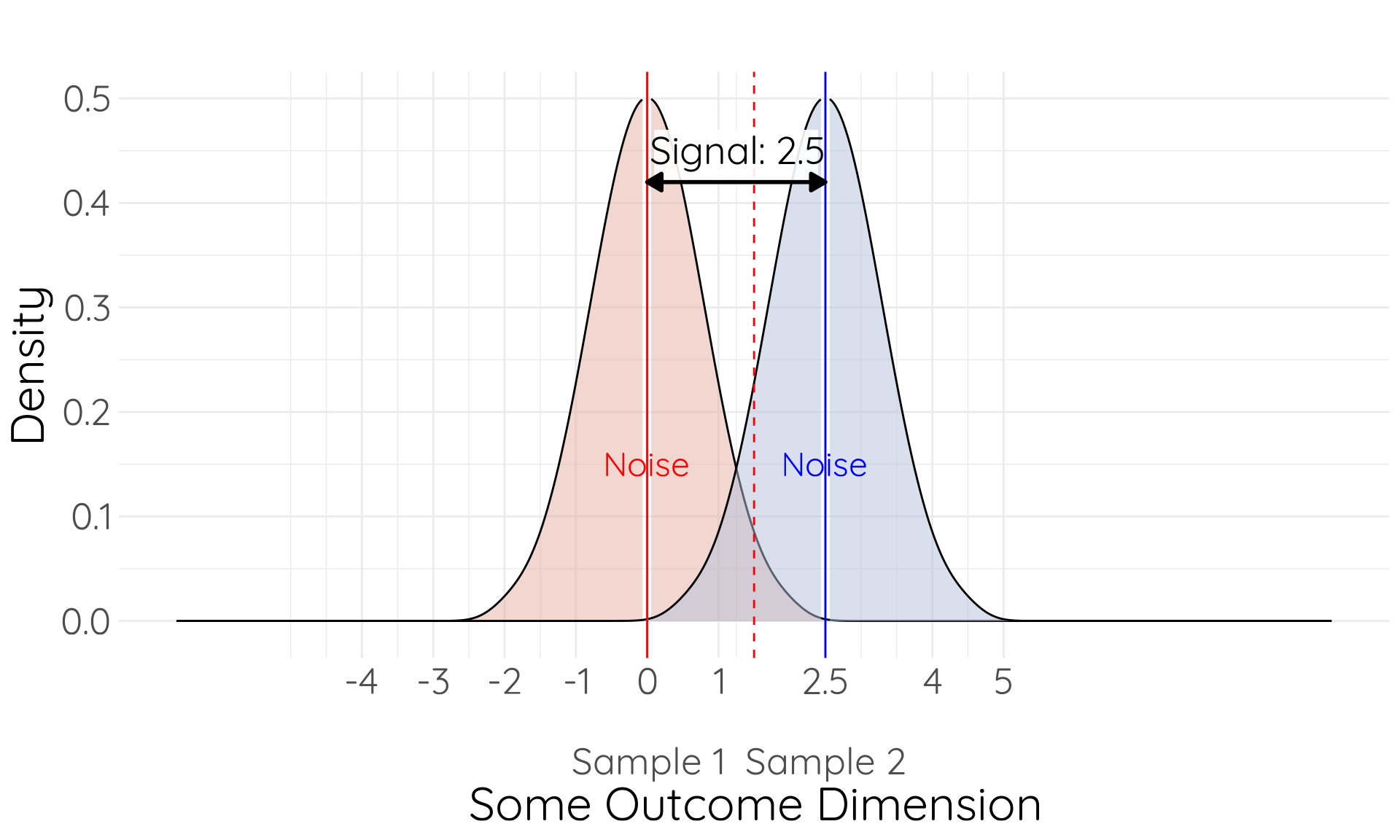
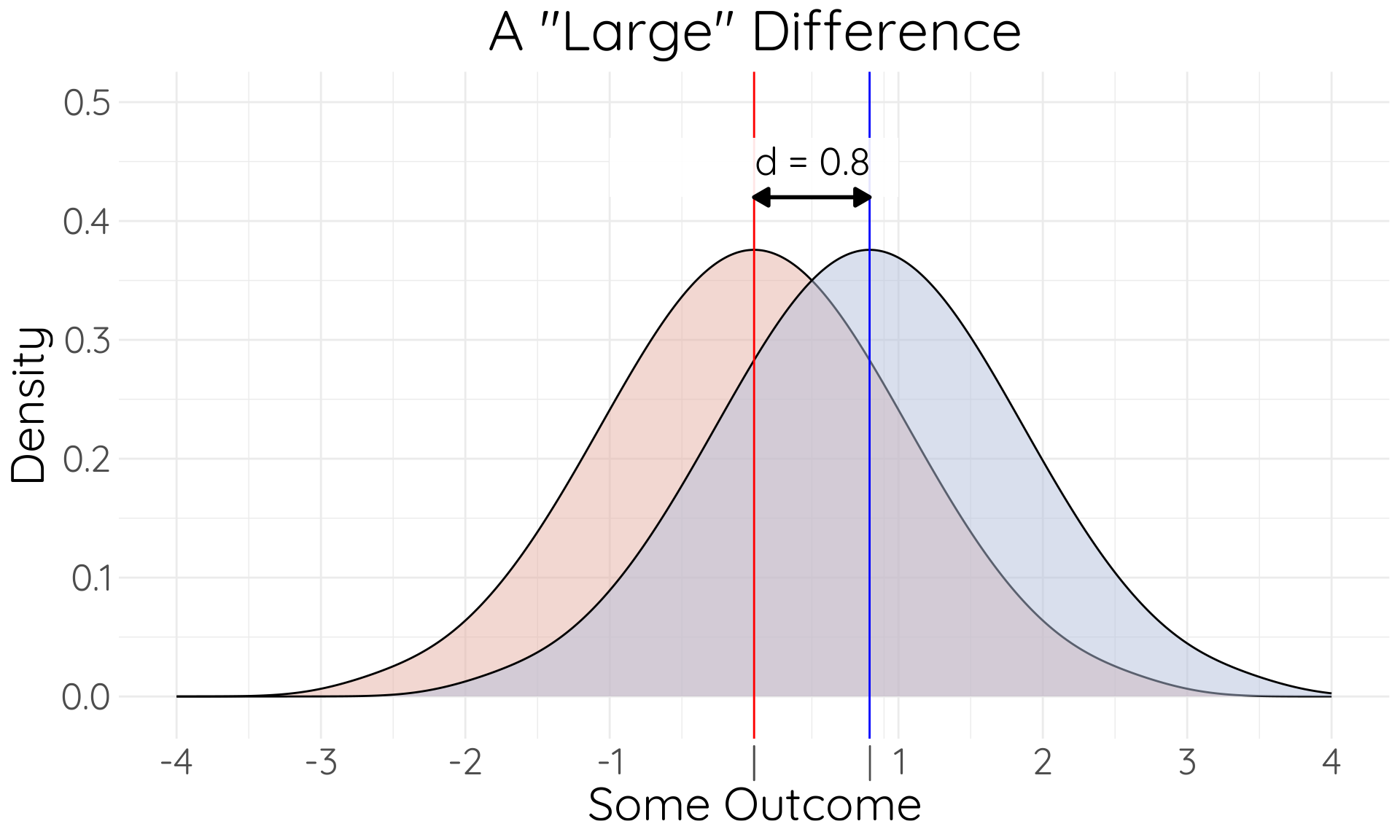
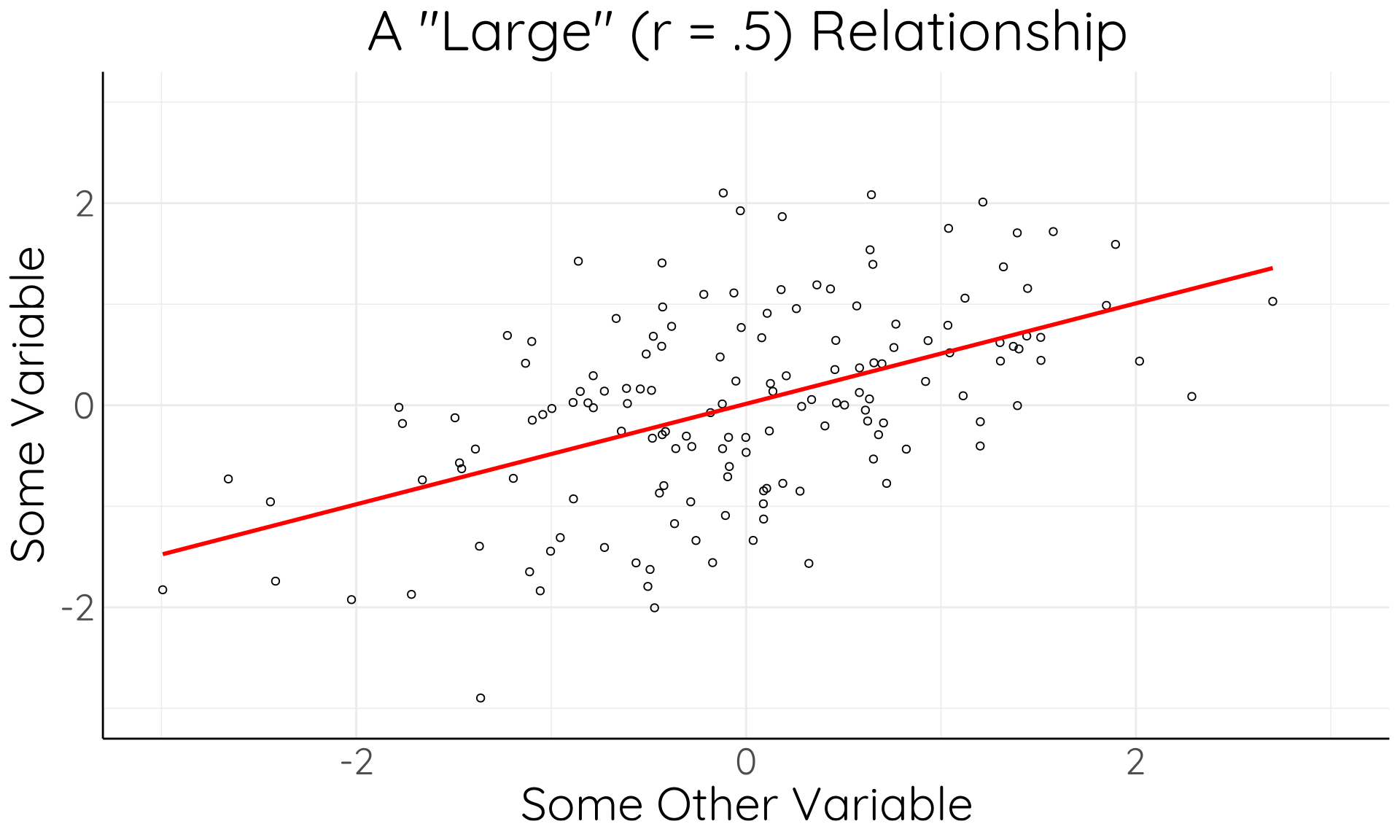
A “Large” Effect
- Large: “must not be so large that their quest by statistical methods is wholly a labor of supererogation,
A “Large” Effect (cont.)
A “Large” Effect (cont.)
A “Large” Effect (end)
- ~53% overlap between two distributions
- 25% of total variance accounted for (~26% for R2)
- Detecting prevalences of 75:25 versus 50:50
- E.g., a mean difference in IQ between “between holders of the
Ph.D. degree and typical college freshmen”
- Or “between college graduates and persons with only a 50-50 chance of passing in an academic high school curriculum” p. 27)
- For significance, would expect to need Ns of:
- t test: 26
- r: 28
Why Use Effect Size?
- “Being forced to think in more exact terms than demanded by the
Fisherian alternative ([i.e., that an effect size] is any nonzero value)
is likely to prove salutary.
[Researchers] can call upon theory for some help in answering the question and on [their] critical assessment of prior research in the area for further help [in using effect size to make nuanced decisions about data].” (p. 12)
And isn’t that the point of research in the first place?